منشوريات
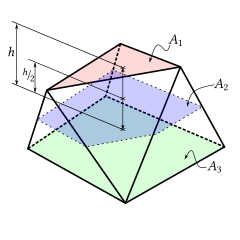
In geometry, a prismatoid is a polyhedron whose vertices all lie in two parallel planes. Its lateral faces can be trapezoids or triangles.[1] If both planes have the same number of vertices, and the lateral faces are either parallelograms or trapezoids, it is called a prismoid.[2]
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
الحجم
If the areas of the two parallel faces are A1 and A3, the cross-sectional area of the intersection of the prismatoid with a plane midway between the two parallel faces is A2, and the height (the distance between the two parallel faces) is h, then the volume of the prismatoid is given by[3]
عائلات المنشوريات
| Pyramids | Wedges | Parallelepipeds | Prisms | Antiprisms | Cupolae | Frusta | ||
|---|---|---|---|---|---|---|---|---|

|

|
|

|

|
| |||
Families of prismatoids include:
- Pyramids, in which one plane contains only a single point;
- Wedges, in which one plane contains only two points;
- Prisms, whose polygons in each plane are congruent and joined by rectangles or parallelograms;
- Antiprisms, whose polygons in each plane are congruent and joined by an alternating strip of triangles;
- Star antiprisms;
- Cupolae, in which the polygon in one plane contains twice as many points as the other and is joined to it by alternating triangles and rectangles;
- Frusta obtained by truncation of a pyramid;
- Quadrilateral-faced hexahedral prismatoids:
- Parallelepipeds – six parallelogram faces
- Rhombohedrons – six rhombus faces
- Trigonal trapezohedra – six congruent rhombus faces
- Cuboids – six rectangular faces
- Quadrilateral frusta – an apex-truncated square pyramid
- Cube – six square faces
الأبعاد الأعلى
In general, a polytope is prismatoidal if its vertices exist in two hyperplanes. For example, in four dimensions, two polyhedra can be placed in two parallel 3-spaces, and connected with polyhedral sides.
المراجع
- ^ Kern, William F.; Bland, James R. (1938). Solid Mensuration with proofs. p. 75.
- ^ Alsina, Claudi; Nelsen, Roger B. (2015). A Mathematical Space Odyssey: Solid Geometry in the 21st Century. The Mathematical Association of America. p. 85. ISBN 9780883853580.
- ^ Meserve, B. E.; Pingry, R. E. (1952). "Some Notes on the Prismoidal Formula". The Mathematics Teacher. 45 (4): 257–263. JSTOR 27954012.