السباعي

Regular heptagon
A regular heptagon
النوع	مضلع منتظم
الأضلاع والرؤوس	{{{p7 جانب}}}
رمز شلفلي	{{{p7-شلفلي}}}
مخططات كوكستر-دنكن
مجموعة التماثل	Dihedral (D7), order 2×7
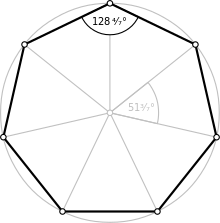
الزاوية الداخلية (الدرجات)	≈128.571°
الخصائص	Convex, cyclic, equilateral, isogonal, isotoxal

السباعي Heptagon المنتظم: هو مضلع محدب يتكون من اتحاد سبعة قطع مستقيمة متساوية الطول، وزواياه السبعة متساوية القياس. ومن المعلوم أيضا أنه يستحيل إنشاء السياعي المنتظم باستخدام المسطرة و الفرجار.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

 السباعي المنتظم

regular heptagon, in which all sides and all angles are equal, has internal angles of 5π/7 radians (128⁴⁄₇ degrees). Its Schläfli symbol is {7}.

 Area

The area (A) of a regular heptagon of side length a is given by:

$A={\frac {7}{4}}a^{2}\cot {\frac {\pi }{7}}\simeq 3.634a^{2}.$

This can be seen by subdividing the unit-sided heptagon into seven triangular "pie slices" with vertices at the center and at the heptagon's vertices, and then halving each triangle using the apothem as the common side. The apothem is half the cotangent of $\pi /7,$ and the area of each of the 14 small triangles is one-fourth of the apothem.

The exact algebraic expression, starting from the cubic polynomial x³ + x² − 2x − 1 (one of whose roots is $2\cos {\tfrac {2\pi }{7}}$) is given in complex numbers by:

$A={\frac {a^{2}}{4}}{\sqrt {{\frac {7}{3}}\left(35+2{\sqrt[{3}]{14^{2}(13-3{\sqrt {-3}})}}+2{\sqrt[{3}]{14^{2}(13+3{\sqrt {-3}})}}\right)}},$

in which the imaginary parts offset each other leaving a real-valued expression. This expression cannot be algebraically rewritten without complex components, since the indicated cubic function is casus irreducibilis.

The area of a regular heptagon inscribed in a circle of radius R is ${\tfrac {7R^{2}}{2}}\sin {\tfrac {2\pi }{7}},$ while the area of the circle itself is $\pi R^{2};$ thus the regular heptagon fills approximately 0.8710 of its circumscribed circle.

 الإنشاء

As 7 is a Pierpont prime but not a Fermat prime, the regular heptagon is not constructible with compass and straightedge but is constructible with a marked ruler and compass. It is the smallest regular polygon with this property. This type of construction is called a neusis construction. It is also constructible with compass, straightedge and angle trisector. The impossibility of straightedge and compass construction follows from the observation that $\scriptstyle {2\cos {\tfrac {2\pi }{7}}\approx 1.247}$ is a zero of the irreducible cubic x³ + x² − 2x − 1. Consequently, this polynomial is the minimal polynomial of 2cos(^2π⁄₇), whereas the degree of the minimal polynomial for a constructible number must be a power of 2.

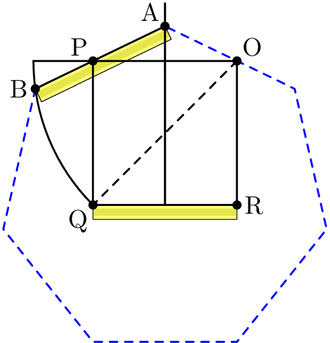A neusis construction of the interior angle in a regular heptagon.

An animation from a neusis construction with radius of circumcircle ${\overline {OA}}=6$, according to Andrew M. Gleason based on the angle trisection by means of the Tomahawk, pause at the end of 30 s.

Heptagon with given side length:
An animation from a neusis construction with marked ruler, according to David Johnson Leisk (Crockett Johnson), pause at the end of 30 s.

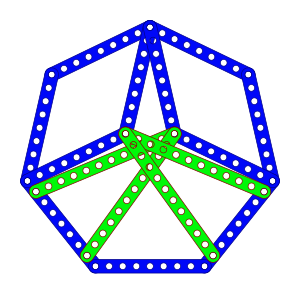
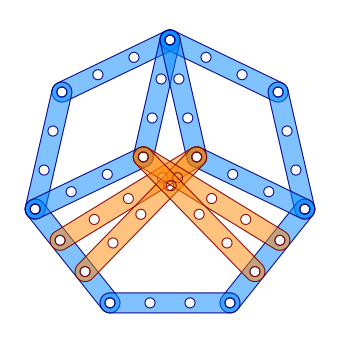
Gerard 't Hooft shows a regular heptagon made of only 15 strips of Meccano with bars sizes 8 and 11.

Meccano heptagon 2

The construction includes two isosceles triangles which hold the rest of bars fixed. The regular heptagon's side a, the shorter isosceles triangle side e, and the longer isosceles triangle side d satisfy

$7a^{2}+e^{2}=4d^{2}$

$d>a>e>0$

The formula is derived from this Heptagonal triangle formula:

$\sin A-\sin B-\sin C=-{\frac {\sqrt {7}}{2}}$

Small possible heptagons constructions:

Heptagon	a	d	e
1	3	4	1
2	8	11	6
3	33	46	29
4	40	53	6
5	55	74	27

The smallest meccano heptagon 1:

 Approximation

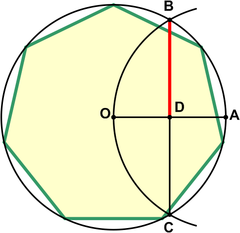
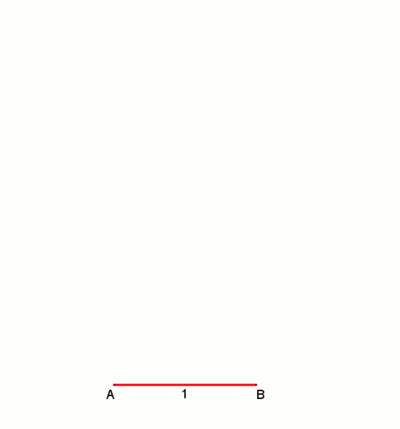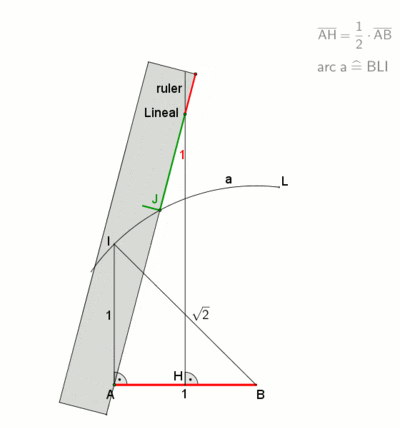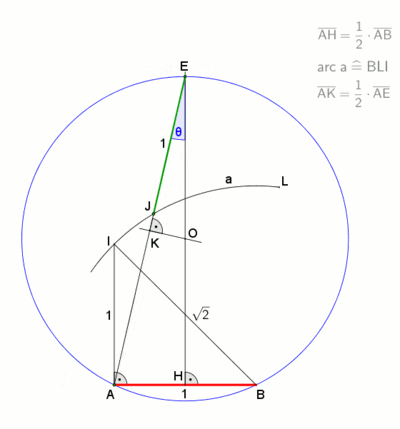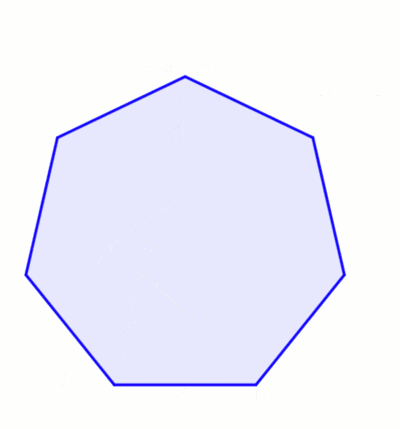
An approximation for practical use with an error of about 0.2% is shown in the drawing. It is attributed to Albrecht Dürer. Let A lie on the circumference of the circumcircle. Draw arc BOC. Then $\scriptstyle {BD={1 \over 2}BC}$ gives an approximation for the edge of the heptagon.

This approximation uses $\scriptstyle {{\sqrt {3}} \over 2}\approx 0.86603$ for the side of the heptagon inscribed in the unit circle while the exact value is $\scriptstyle 2\sin {\pi \over 7}\approx 0.86777$.

Example to illustrate the error:
At a circumscribed circle radius r = 1 m, the absolute error of the 1st side would be approximately -1.7 mm

Meccano approximated heptagon. Bar sizes are 20, 36 and 45.

A meccano approximation construction can be made with eleven bars of sizes 20, 36 and 45. These values leave an error around 0.1%.

 Symmetry

Symmetries of a regular heptagon. Vertices are colored by their symmetry positions. Blue mirror lines are drawn through vertices and edges. Gyration orders are given in the center.

 Diagonals and heptagonal triangle

a=red, b=blue, c=green lines

The regular heptagon's side a, shorter diagonal b, and longer diagonal c, with a<b<c, satisfy^{:Lemma 1}

$a^{2}=c(c-b),$

$b^{2}=a(c+a),$

$c^{2}=b(a+b),$

${\frac {1}{a}}={\frac {1}{b}}+{\frac {1}{c}}$ (the optic equation)

and hence

$ab+ac=bc,$

and^{:Coro. 2}

$b^{3}+2b^{2}c-bc^{2}-c^{3}=0,$

$c^{3}-2c^{2}a-ca^{2}+a^{3}=0,$

$a^{3}-2a^{2}b-ab^{2}+b^{3}=0,$

Thus –b/c, c/a, and a/b all satisfy the cubic equation $t^{3}-2t^{2}-t+1=0.$ However, no algebraic expressions with purely real terms exist for the solutions of this equation, because it is an example of casus irreducibilis.

The approximate lengths of the diagonals in terms of the side of the regular heptagon are given by

$b\approx 1.80193\cdot a,\qquad c\approx 2.24698\cdot a.$

We also have

$b^{2}-a^{2}=ac,$

$c^{2}-b^{2}=ab,$

$a^{2}-c^{2}=-bc,$

and

${\frac {b^{2}}{a^{2}}}+{\frac {c^{2}}{b^{2}}}+{\frac {a^{2}}{c^{2}}}=5.$

A heptagonal triangle has vertices coinciding with the first, second, and fourth vertices of a regular heptagon (from an arbitrary starting vertex) and angles $\pi /7,2\pi /7,$ and $4\pi /7.$ Thus its sides coincide with one side and two particular diagonals of the regular heptagon.

  Star heptagons

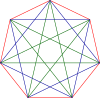
Two kinds of star heptagons (heptagrams) can be constructed from regular heptagons, labeled by Schläfli symbols {7/2}, and {7/3}, with the divisor being the interval of connection.

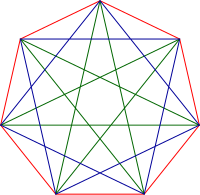
Blue, {7/2} and green {7/3} star heptagons inside a red heptagon.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

 Empirical examples

Geometry problem of the surface of a heptagon divided into triangles, on a clay tablet belonging to a school for scribes; Susa, first half of the 2nd millennium BCE

 Graphs

The K₇ complete graph is often drawn as a regular heptagon with all 21 edges connected. This graph also represents an orthographic projection of the 7 vertices and 21 edges of the 6-simplex. The regular skew polygon around the perimeter is called the petrie polygon.

6-simplex (6D)

 Heptagon in nature

• 

  Cactus

 See also

• Heptagram
• Polygon

 External links

ابحث عن heptagon في
قاموس المعرفة.

• Definition and properties of a heptagon With interactive animation
• Heptagon according Johnson
• Another approximate construction method
• Polygons – Heptagons
• Recently discovered and highly accurate approximation for the construction of a regular heptagon.
• Heptagon, an approximating construction as an animation
• A heptagon with a given side, an approximating construction as an animation