نظام جذري
| Group theory → زُمـَر لي زُمـَر لي |
|---|
 |
في الرياضيات، النظام الجذري Root system هو تشكيل من الأشعة في الفضاء الإقليدي ، يحقق خواصا هندسية معينة، هذا المصطلح ذو أهمية خاصة نظرية زمرة لي، خصوصاً تبويب وتمثيل نظرية جبر لي شبه البسيط. Since Lie groups (and some analogues such as algebraic groups) and Lie algebras have become important in many parts of mathematics during the twentieth century, the apparently special nature of root systems belies the number of areas in which they are applied. Further, the classification scheme for root systems, by Dynkin diagrams, occurs in parts of mathematics with no overt connection to Lie theory (such as singularity theory). Finally, root systems are important for their own sake, as in spectral graph theory.[1]
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
تعريفات وأمثلة
تعريف
Let E be a finite-dimensional Euclidean vector space, with the standard Euclidean inner product denoted by . A root system in E is a finite set of non-zero vectors (called roots) that satisfy the following conditions:[2][3]
- The roots span E.
- The only scalar multiples of a root that belong to are itself and .
- For every root , the set is closed under reflection through the hyperplane perpendicular to .
- (Integrality) If and are roots in , then the projection of onto the line through is an integer or half-integer multiple of .
An equivalent way of writing conditions 3 and 4 is as follows:
- For any two roots , the set contains the element
- For any two roots , the number is an integer.
Some authors only include conditions 1–3 in the definition of a root system.[4] In this context, a root system that also satisfies the integrality condition is known as a crystallographic root system.[5] Other authors omit condition 2; then they call root systems satisfying condition 2 reduced.[6] In this article, all root systems are assumed to be reduced and crystallographic.
In view of property 3, the integrality condition is equivalent to stating that β and its reflection σα(β) differ by an integer multiple of α. Note that the operator
defined by property 4 is not an inner product. It is not necessarily symmetric and is linear only in the first argument.
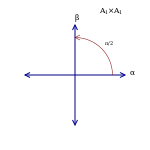
|
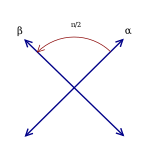
|
| Root system |
Root system |
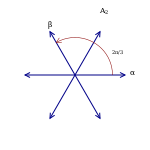
|

|
| Root system |
Root system |
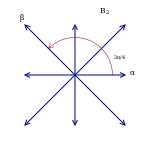
|
|
| Root system |
Root system |
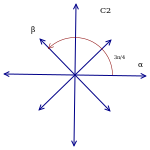
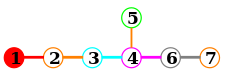
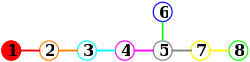
The rank of a root system Φ is the dimension of E. Two root systems may be combined by regarding the Euclidean spaces they span as mutually orthogonal subspaces of a common Euclidean space. A root system which does not arise from such a combination, such as the systems A2, B2, and G2 pictured to the right, is said to be irreducible.
Two root systems (E1, Φ1) and (E2, Φ2) are called isomorphic if there is an invertible linear transformation E1 → E2 which sends Φ1 to Φ2 such that for each pair of roots, the number is preserved.[7]
The root lattice of a root system Φ is the Z-submodule of E generated by Φ. It is a lattice in E.
Weyl group
Weyl chambers and the Weyl group
E6, E7, E8
 72 vertices of 122 represent the root vectors of E6 (Green nodes are doubled in this E6 Coxeter plane projection) |
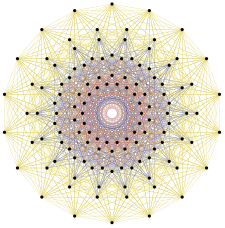 126 vertices of 231 represent the root vectors of E7 |
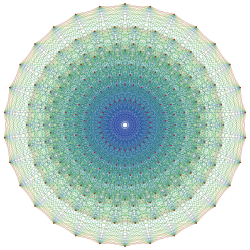 240 vertices of 421 represent the root vectors of E8 |
|
|
|
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
انظر أيضاً
- ADE classification
- Affine root system
- Coxeter–Dynkin diagram
- Coxeter group
- Coxeter matrix
- Dynkin diagram
- root datum
- Semisimple Lie algebra
- Weights in the representation theory of semisimple Lie algebras
- Root system of a semi-simple Lie algebra
- Weyl group
الهامش
- ^ "Graphs with least eigenvalue −2; a historical survey and recent developments in maximal exceptional graphs". Linear Algebra and its Applications. 356: 189–210. doi:10.1016/S0024-3795(02)00377-4.
- ^ Bourbaki, Ch.VI, Section 1
- ^ Humphreys (1972), p.42
- ^ Humphreys (1992), p.6
- ^ Humphreys (1992), p.39
- ^ Humphreys (1992), p.41
- ^ Humphreys (1972), p.43
المراجع
- Adams, J.F. (1983), Lectures on Lie groups, University of Chicago Press, ISBN 0-226-00530-5
- Bourbaki, Nicolas (2002), Lie groups and Lie algebras, Chapters 4–6 (translated from the 1968 French original by Andrew Pressley), Elements of Mathematics, Springer-Verlag, ISBN 3-540-42650-7. The classic reference for root systems.
- Bourbaki, Nicolas (1998). Elements of the History of Mathematics. Springer. ISBN 3540647678.
- A.J. Coleman (Summer 1989), "The greatest mathematical paper of all time", The Mathematical Intelligencer 11 (3): 29–38, doi:
- Hall, Brian C. (2015), Lie groups, Lie algebras, and representations: An elementary introduction, Graduate Texts in Mathematics, 222 (2nd ed.), Springer, ISBN 978-3319134666
- Humphreys, James (1992). Reflection Groups and Coxeter Groups. Cambridge University Press. ISBN 0521436133.
- Humphreys, James (1972). Introduction to Lie algebras and Representation Theory. Springer. ISBN 0387900535.
- Killing, Die Zusammensetzung der stetigen endlichen Transformationsgruppen Mathematische Annalen, Part 1: Volume 31, Number 2 June 1888, Pages 252-290 doi:10.1007/BF01211904; Part 2[dead link]: Volume 33, Number 1 March 1888, Pages 1–48 doi:10.1007/BF01444109; Part3: Volume 34, Number 1 March 1889, Pages 57–122 doi:10.1007/BF01446792; Part 4[dead link]: Volume 36, Number 2 June 1890,Pages 161-189 doi:10.1007/BF01207837
- Kac, Victor G. (1994), Infinite dimensional Lie algebras.
- Springer, T.A. (1998). Linear Algebraic Groups, Second Edition. Birkhäuser. ISBN 0817640215.
للاستزادة
- Dynkin, E. B. The structure of semi-simple algebras. (بالروسية) Uspehi Matem. Nauk (N.S.) 2, (1947). no. 4(20), 59–127.




















