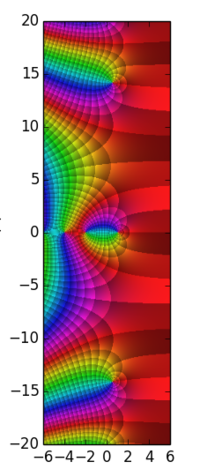
اقتران ريمان الزائي
| اقتران ريمان الزائي | |
|---|---|
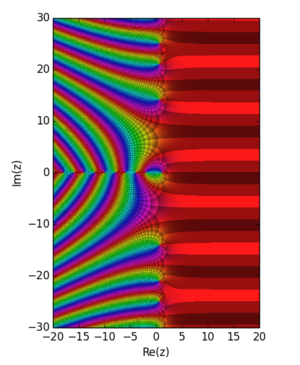 | |
| Basic features | |
| Domain | |
| Codomain | |
| Specific values | |
| At zero | |
| Limit to +∞ | |
| Value at | |
| Value at | |
| Value at | |
الدالة زيتا (اِقْتِرانُ ريمان الزَّائِيُّ حسب مجمع اللغة العربية بالقاهرة) دالة خاصّة لها أهمية عظيمة في نظرية الأعداد. تعريفها المشهور الصالح لأجل
يمكن تعريفها بصيغ أخرى عدبدة نخص بالذكر منها جداء أويلر
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
التعريف
صيغة الدالة للأعداد الزوجية
هذه الصيغة تنسب لأويلر، وهي تعطي قيمة ζ(2k) للأعداد الزوجية:
حيث B2k هي أعداد بيرنولي.
و هذه بعض القيم:
ζ(2) = π2/6, ζ(4) = π4/90, ζ(6) = π6/945, ζ(8) = π8/9450
أما بالنسبة للأعداد الفردية, فلا توجد صيغة لحساب زيتا. فقط نعرف قيمة 3 التي هي: ζ(3) = 1,2020569 ،
انظر أيضاً
- 1 + 2 + 3 + 4 + ···
- Arithmetic zeta function
- Generalized Riemann hypothesis
- Lehmer pair
- Particular values of Riemann zeta function
- Prime zeta function
- Riemann Xi function
- Renormalization
- Riemann–Siegel theta function
- ZetaGrid
الهامش
- ^ "Jupyter Notebook Viewer". Nbviewer.ipython.org. Retrieved 2017-01-04.
المراجع
- قالب:Dlmf
- Borwein, Jonathan; Bradley, David M.; Crandall, Richard (2000). "Computational Strategies for the Riemann Zeta Function" (PDF). J. Comp. App. Math. 121 (1–2): 247–296. Bibcode:2000JCoAM.121..247B. doi:10.1016/S0377-0427(00)00336-8.
- Cvijović, Djurdje; Klinowski, Jacek (2002). "Integral Representations of the Riemann Zeta Function for Odd-Integer Arguments". J. Comp. App. Math. 142 (2): 435–439. Bibcode:2002JCoAM.142..435C. doi:10.1016/S0377-0427(02)00358-8. MR 1906742.
- Cvijović, Djurdje; Klinowski, Jacek (1997). "Continued-fraction expansions for the Riemann zeta function and polylogarithms". Proc. Amer. Math. Soc. 125 (9): 2543–2550. doi:10.1090/S0002-9939-97-04102-6.
- Edwards, H. M. (1974). Riemann's Zeta Function. Academic Press. ISBN 0-486-41740-9. Has an English translation of Riemann's paper.
- Hadamard, Jacques (1896). "Sur la distribution des zéros de la fonction ζ(s) et ses conséquences arithmétiques". Bulletin de la Société Mathématique de France. 14: 199–220. doi:10.24033/bsmf.545.
- Hardy, G. H. (1949). Divergent Series. Clarendon Press, Oxford.
- Hasse, Helmut (1930). "Ein Summierungsverfahren für die Riemannsche ζ-Reihe". Math. Z. 32: 458–464. doi:10.1007/BF01194645. MR 1545177. S2CID 120392534. (Globally convergent series expression.)
- Ivic, A. (1985). The Riemann Zeta Function. John Wiley & Sons. ISBN 0-471-80634-X.
- Motohashi, Y. (1997). Spectral Theory of the Riemann Zeta-Function. Cambridge University Press. ISBN 0521445205.
- Karatsuba, A. A.; Voronin, S. M. (1992). The Riemann Zeta-Function. Berlin: W. de Gruyter.
- Mező, István; Dil, Ayhan (2010). "Hyperharmonic series involving Hurwitz zeta function". Journal of Number Theory. 130 (2): 360–369. doi:10.1016/j.jnt.2009.08.005. hdl:2437/90539. MR 2564902.
- Montgomery, Hugh L.; Vaughan, Robert C. (2007). Multiplicative number theory. I. Classical theory. Cambridge tracts in advanced mathematics. Vol. 97. Cambridge University Press. Ch. 10. ISBN 978-0-521-84903-6.
- Newman, Donald J. (1998). Analytic number theory. Graduate Texts in Mathematics. Vol. 177. Springer-Verlag. Ch. 6. ISBN 0-387-98308-2.
- Raoh, Guo (1996). "The Distribution of the Logarithmic Derivative of the Riemann Zeta Function". Proceedings of the London Mathematical Society. s3–72: 1–27. arXiv:1308.3597. doi:10.1112/plms/s3-72.1.1.
- Riemann, Bernhard (1859). "Über die Anzahl der Primzahlen unter einer gegebenen Grösse". Monatsberichte der Berliner Akademie.. In Gesammelte Werke, Teubner, Leipzig (1892), Reprinted by Dover, New York (1953).
- Sondow, Jonathan (1994). "Analytic continuation of Riemann's zeta function and values at negative integers via Euler's transformation of series" (PDF). Proc. Amer. Math. Soc. 120 (2): 421–424. doi:10.1090/S0002-9939-1994-1172954-7.
- Titchmarsh, E. C. (1986). Heath-Brown (ed.). The Theory of the Riemann Zeta Function (2nd rev. ed.). Oxford University Press.
- Whittaker, E. T.; Watson, G. N. (1927). A Course in Modern Analysis (4th ed.). Cambridge University Press. Ch. 13.
- Zhao, Jianqiang (1999). "Analytic continuation of multiple zeta functions". Proc. Amer. Math. Soc. 128 (5): 1275–1283. doi:10.1090/S0002-9939-99-05398-8. MR 1670846.
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
وصلات خارجية
- Hazewinkel, Michiel, ed. (2001), "Zeta-function", Encyclopaedia of Mathematics, Kluwer Academic Publishers, ISBN 978-1556080104
- Riemann Zeta Function, in Wolfram Mathworld — an explanation with a more mathematical approach
- Tables of selected zeros
- Prime Numbers Get Hitched A general, non-technical description of the significance of the zeta function in relation to prime numbers.
- X-Ray of the Zeta Function Visually oriented investigation of where zeta is real or purely imaginary.
- Formulas and identities for the Riemann Zeta function functions.wolfram.com
- Riemann Zeta Function and Other Sums of Reciprocal Powers, section 23.2 of Abramowitz and Stegun
- Frenkel, Edward. "Million Dollar Math Problem" (video). Brady Haran. Retrieved 11 March 2014.
- Mellin transform and the functional equation of the Riemann Zeta function—Computational examples of Mellin transform methods involving the Riemann Zeta Function