نجمة عشارية

Regular decagram
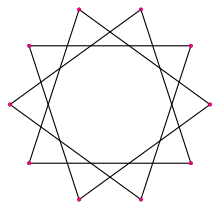A regular decagram
النوع	مضلع منتظم
الأضلاع والرؤوس	10
رمز شلفلي	{10/3} t{5/3}
مخططات كوكستر-دنكن	{{{p10/3-CD2}}}
مجموعة التماثل	Dihedral (D10)
الزاوية الداخلية (الدرجات)	72°
الخصائص	نجم، cyclic, equilateral, isogonal, isotoxal

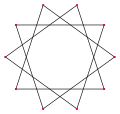
في الهندسة، النجمة العشارية decagram، هي مضلع نجمي بعشر رؤوس. وهناك نجمة عشرية منتظمة واحدة، تضم رؤوس مشلع عشري منتظم، ولكن متصلة بكل ثالث رأس. رمز شلفلي لها هو {10/3}.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

 نجمة عشارية منتظمة

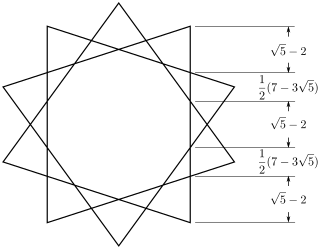
For a regular decagram with unit edge lengths, the proportions of the crossing points on each edge are as shown below.

 التطبيقات

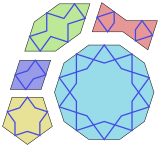
Decagrams have been used as one of the decorative motifs in girih tiles.

 أشكال ذات صلة

A regular decagram is a 10-sided polygram, represented by symbol {10/n}, containing the same vertices as regular decagon. Only one of these polygrams, {10/3} (connecting every third point), forms a regular star polygon, but there are also three ten-vertex polygrams which can be interpreted as regular compounds:

• {10/5} is a compound of five degenerate digons 5{2}
• {10/4} is a compound of two pentagrams 2{5/2}
• {10/2} is a compound of two pentagons 2{5}.

الشكل	Convex	المركب	المضلع النجمي	المركـّبات
Image
Symbol	{10/1} = {10}	{10/2} = 2{5}	{10/3}	{10/4} = 2{5/2}	{10/5} = 5{2}

Isogonal truncations of pentagon and pentagram

Quasiregular	Isogonal		Quasiregular Double covering
t{5} = {10}			t{5/4} = {10/4} = 2{5/2}
t{5/3} = {10/3}			t{5/2} = {10/2} = 2{5}

 رموز

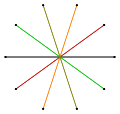
{10/2} or 2{5} is a compound of 2 pentagons.

{10/4} or 2{5/2} is a compound of 2 pentagrams.

 نجوم عشارية أخرى

 انظر أيضا

• List of regular polytopes and compounds#Stars