مضلع قابل للإنشاء
في الرياضيات، المضلع القابل للإنشاء هو مضلع منتظم يكون من الممكن إنشاؤه باستخدم إنشاءات الفرجار والمسطرة.
مثلاً، المخمس المنتظم هو مضلع قابل للإنشاء، بينما السباعي المنتظم هو مضلع غير قابل للإنشاء.
شروط قابلية الإنشاء
Some regular polygons are easy to construct with compass and straightedge; others are not. The ancient Greek mathematicians knew how to construct a regular polygon with 3, 4, or 5 sides,[1] and they knew how to construct a regular polygon with double the number of sides of a given regular polygon.[1] This led to the question being posed: is it possible to construct all regular polygons with compass and straightedge? If not, which n-gons (that is polygons with n edges) are constructible and which are not?
Carl Friedrich Gauss proved the constructibility of the regular 17-gon in 1796. Five years later, he developed the theory of Gaussian periods in his Disquisitiones Arithmeticae. This theory allowed him to formulate a sufficient condition for the constructibility of regular polygons. Gauss stated without proof that this condition was also necessary, but never published his proof. A full proof of necessity was given by Pierre Wantzel in 1837. The result is known as the Gauss–Wantzel theorem:
- A regular n-gon (that is, a polygon with n sides) can be constructed with compass and straightedge if and only if n is the product of a power of 2 and any number of distinct Fermat primes (including none).
(A Fermat prime is a prime number of the form )
In order to reduce a geometric problem to a problem of pure number theory, the proof uses the fact that a regular n-gon is constructible if and only if the cosine, , is a constructible number—that is, can be written in terms of the four basic arithmetic operations and the extraction of square roots. Equivalently, a regular n-gon is constructible if any root of the nth cyclotomic polynomial is constructible.
نتائج مفصلة بنظرية گاوس
Restating the Gauss-Wantzel theorem:
- A regular n-gon is constructible with straightedge and compass if and only if n = 2kp1p2...pt where k and t are non-negative integers, and the pi's (when t > 0) are distinct Fermat primes.
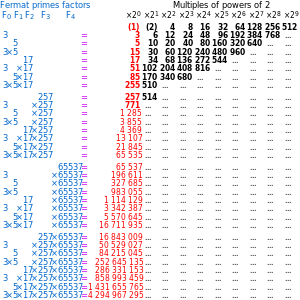
The five known Fermat primes are:
Since there are 31 combinations of anywhere from one to five Fermat primes, there are 31 known constructible polygons with an odd number of sides.
The next twenty-eight Fermat numbers, F5 through F32, are known to be composite.[2]
Thus a regular n-gon is constructible if
- n = 3, 4, 5, 6, 8, 10, 12, 15, 16, 17, 20, 24, 30, 32, 34, 40, 48, 51, 60, 64, 68, 80, 85, 96, 102, 120, 128, 136, 160, 170, 192, 204, 240, 255, 256, 257, 272, 320, 340, 384, 408, 480, 510, 512, 514, 544, 640, 680, 768, 771, 816, 960, 1020, 1024, 1028, 1088, 1280, 1285, 1360, 1536, 1542, 1632, 1920, 2040, 2048, ... (المتتالية A003401 في OEIS),
while a regular n-gon is not constructible with compass and straightedge if
- n = 7, 9, 11, 13, 14, 18, 19, 21, 22, 23, 25, 26, 27, 28, 29, 31, 33, 35, 36, 37, 38, 39, 41, 42, 43, 44, 45, 46, 47, 49, 50, 52, 53, 54, 55, 56, 57, 58, 59, 61, 62, 63, 65, 66, 67, 69, 70, 71, 72, 73, 74, 75, 76, 77, 78, 79, 81, 82, 83, 84, 86, 87, 88, 89, 90, 91, 92, 93, 94, 95, 97, 98, 99, 100, 101, 103, 104, 105, 106, 107, 108, 109, 110, 111, 112, 113, 114, 115, 116, 117, 118, 119, 121, 122, 123, 124, 125, 126, 127, ... (المتتالية A004169 في OEIS).
الصلة بمثلث پاسكال
Since there are 5 known Fermat primes, we know of 31 numbers that are products of distinct Fermat primes, and hence 31 constructible odd-sided regular polygons. These are 3, 5, 15, 17, 51, 85, 255, 257, 771, 1285, 3855, 4369, 13107, 21845, 65535, 65537, 196611, 327685, 983055, 1114129, 3342387, 5570645, 16711935, 16843009, 50529027, 84215045, 252645135, 286331153, 858993459, 1431655765, 4294967295 (المتتالية A045544 في OEIS). As John Conway commented in The Book of Numbers, these numbers, when written in binary, are equal to the first 32 rows of the modulo-2 Pascal's triangle, minus the top row, which corresponds to a monogon. (Because of this, the 1s in such a list form an approximation to the Sierpiński triangle.) This pattern breaks down after this, as the next Fermat number is composite (4294967297 = 641 × 6700417), so the following rows do not correspond to constructible polygons. It is unknown whether any more Fermat primes exist, and it is therefore unknown how many odd-sided constructible regular polygons exist. In general, if there are q Fermat primes, then there are 2q−1 odd-sided regular constructible polygons.
معرض الرسم

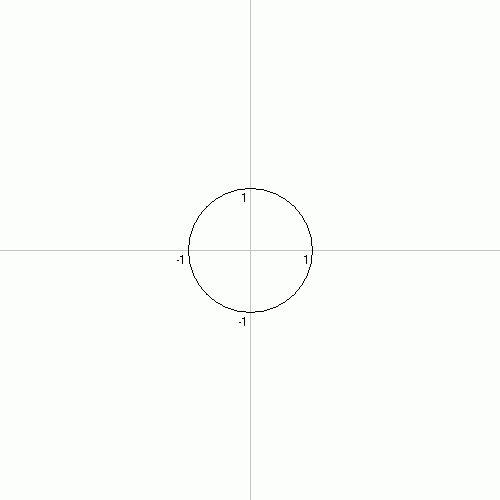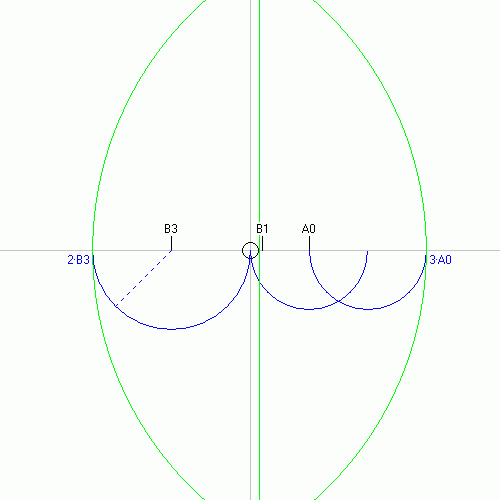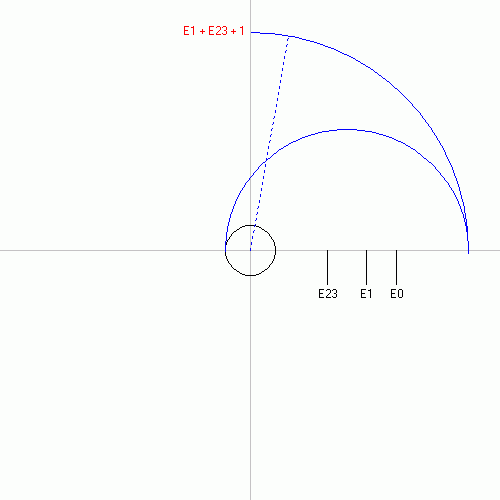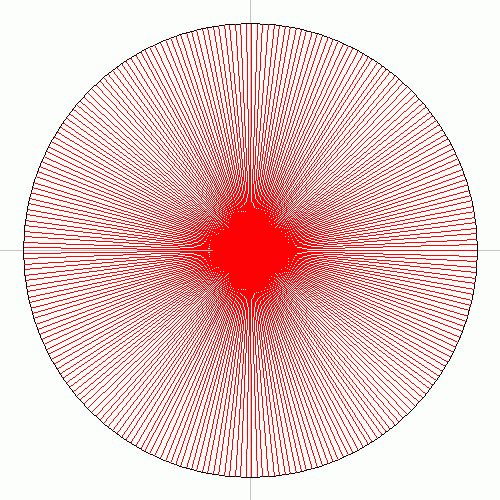
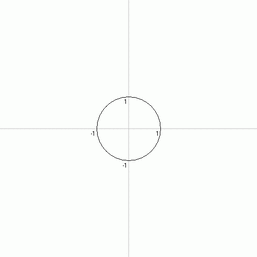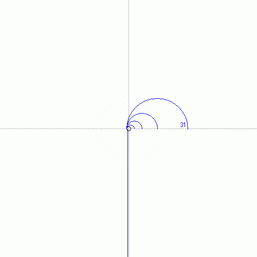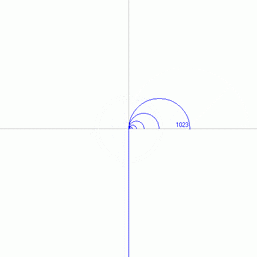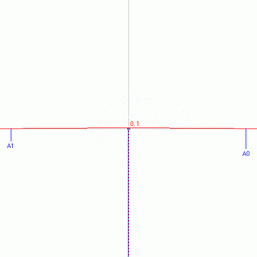
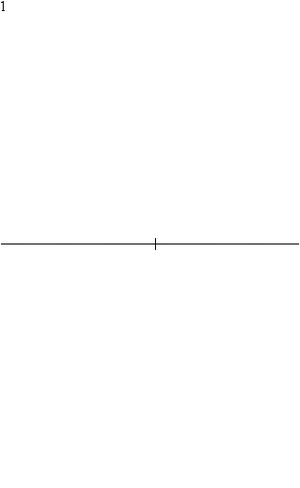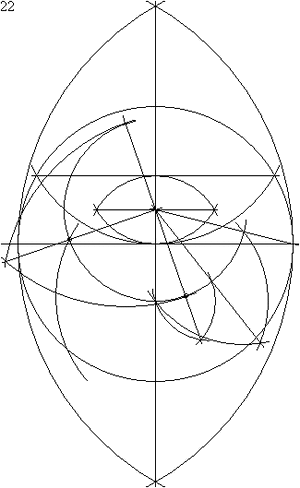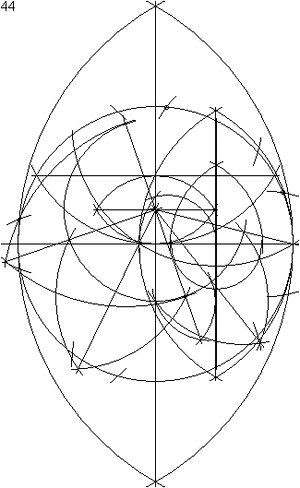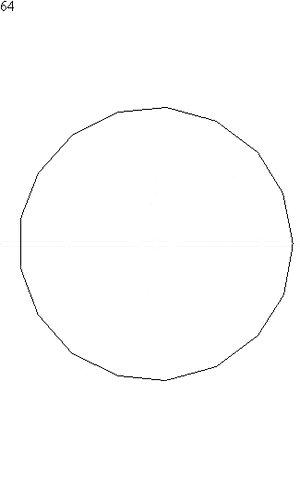
From left to right, constructions of a 15-gon, 17-gon, 257-gon and 65537-gon. Only the first stage of the 65537-gon construction is shown; the constructions of the 15-gon, 17-gon, and 257-gon are given complete.
انظر أيضاً
الهامش
- ^ أ ب Bold, Benjamin. Famous Problems of Geometry and How to Solve Them, Dover Publications, 1982 (orig. 1969).
- ^ Fermat factoring status Archived 2016-02-10 at the Wayback Machine by Wilfrid Keller.
وصلات خارجية
- Duane W. DeTemple (1991). "Carlyle Circles and the Lemoine Simplicity of Polygonal Constructions". The American Mathematical Monthly. 98 (2): 97–108. doi:10.2307/2323939. JSTOR 2323939. MR 1089454.
- Christian Gottlieb (1999). "The Simple and Straightforward Construction of the Regular 257-gon". Mathematical Intelligencer. 21 (1): 31–37. doi:10.1007/BF03024829. MR 1665155.
- Regular Polygon Formulas, Ask Dr. Math FAQ.
- Carl Schick: Weiche Primzahlen und das 257-Eck : eine analytische Lösung des 257-Ecks. Zürich : C. Schick, 2008. ISBN 978-3-9522917-1-9.
- 65537-gon, exact construction for the 1st side, using the Quadratrix of Hippias and GeoGebra as additional aids, with brief description (German)