تصادم مرن

تتصادم جزيئات الهواء عادة تصادما مرنا .

في التصادم المرن تكون طاقة الحركة الكلية بعد التصادم مساوية لطاقة الحركة الكلية قبل التصادم . فالتصادم المرن يحدث فقط عندما لا يتحول أي جزء من طاقة الحركة خلال التصادم إلى طاقة من نوع آخر. وخلال التصادم تتحول طاقة الحركة أولا إلى طاقة وضع مصحوبة بقوة رد فعل طاردة بين الأجسام ، وبعدها تتحول ثانيا إلى طاقة حركة منقسمة على الأجسام بحسب كتلة كل منها . ويعتبر تصادم جزيئات الهواء من جزيئات أكسجين وجزيئات نيتروجين نوعا للتصادم المرن .

ولكن من أجل الحفاظ على دقة القول فعند اصتدام الجزيئات أو الذرات في الحالة الغازية أو السائلة قليلا ما يحدث التصادم المرن المثالي ، فبعض من طاقة الحركة تأخذها بعض الجزيئات كطاقة دورانية أو طاقة اهتزازية بحسب ما لها من إمكانيات ( إمكانيات الجزيئ للحركة الانتقالية ، أو الحركة الدورانية أو الحركة الاهتزازية ، تُسمي درجة حريته degree of freedom ) . ففي حالة الغازات تعتبر نصف التصادمات غير مرنة وهذا معناه أن جزءا من طاقة الحركة الابتدائية تتحول إلى أنواع أخرى من الطاقة .

وفي حياتنا العادية يندر أن يحدث التصادم المرن ، اللهم إلا في حالة اللعب بالكرة أو لعب البلياردو ، فهذا النوع من التصادم يعتبر تصادما مرنا.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

 معادلات التصادم أحادي المحور (التصادم المستقيم)

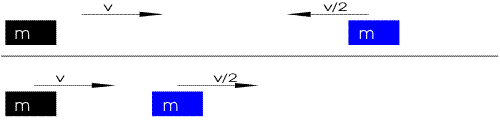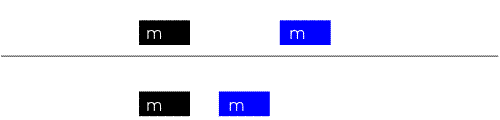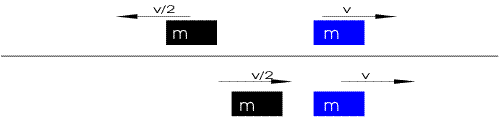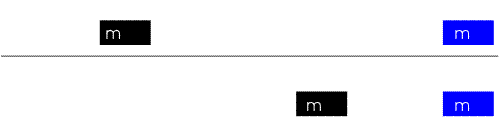
نعني بالتصادم أحادي المحور أن التصادم يحدث هنا على خط مستقيم بدون حدوث أي زوايا تتخذها الأجسام بعد الاصتدام . نفرض أن لدينا جسمان 1 و 2 وكتلة كل منهما m والسرعة u قبل التصادم و v بعد التصادم. فتتساوى طاقة الحركة قبل وبعد التصادم ، وكذلك يتساوى كمية التحرك قبل وبعد التصادم . وطبقا للمعادلة العامة لنيوتن لكمية الحركة والتي تنص على :

m1v1 + m2v2 = 'm1v1 + 'm2v2

إذا ً بالمثل ، فطاقة الحركة قبل وبعد التصادم يجب أن تكون متساويتان ، أي أن:

${\frac {m_{1}u_{1}^{2}}{2}}+{\frac {m_{2}u_{2}^{2}}{2}}={\frac {m_{1}v_{1}^{2}}{2}}+{\frac {m_{2}v_{2}^{2}}{2}}.$

وكمية التحرك الكلية تكون متساوية قبل وبعد التصادم ، أي أن:

$\,\!m_{1}v_{1}+m_{2}v_{2}=m_{1}u_{1}+m_{2}u_{2}.$

يمكن حل تلك المعادلتين للحصول على $\ v_{1}$ و $\ v_{2}$. ولكن ذلك قد يكون مرهقا . ويمكننا تلافي تلك الصعوبة باختيار سرعة أحد الجسمين صفرا ، أي أننا نضع $\ v_{1}$ = 0 أو $\ v_{2}$ = 0 وهذه العملية تعادل تغيير مختبر المشاهدة . ولكن النتيجة لا تتغير بتغير مختبر المشاهدة ، إذ يمكننا بعد الحصول على إحدى السرعات الرجوع إلى مختبرنا الأول لحساب السرعة الثانية .

فبمجرد وضع إحدى السرعتين = 0 يمكننا حل المعادلتين بسهولة ، فنحصل على:

$v_{1}={\frac {u_{1}(m_{1}-m_{2})+2m_{2}u_{2}}{m_{1}+m_{2}}}$ , $v_{2}={\frac {u_{2}(m_{2}-m_{1})+2m_{1}u_{1}}{m_{1}+m_{2}}}$

وبالتالي :

$\ v_{1}=u_{1}$ , $\ v_{2}=u_{2}$.

وإلى هنا تبدو تلك المعادلة الأخيرة كما لو لم يحدث تصادم على الإطلاق ، ولكن لنتريث قليلا ،

وعلى سبيل المثال، إذا كانت لدينا كرتان مختلفتي الكتلة Ball 1 و Ball 2 ومختلفتي السرعة ، قبل التصادم:

Ball 1: mass = 3 kg, v = 4 m/s

Ball 2: mass = 5 kg, v = −6 m/s

وبعد التصادم :

Ball 1: v = −8.5 m/s

Ball 2: v = 1.5 m/s

(ملحوظة : السرعة من القيم المتجهة . لهذا إذا تحركت الأولي من اليمين إلي السار فتكون القيمة المتجهة لسرعتها موجبة ، أما الكرة التي تتحرك من اليسار إلي اليمين فتكون سرعتها المتجهة سالبة.)

نستخلص من القيم قبل التصادم وبعده المعادلة :

$\ v_{1}-v_{2}=u_{2}-u_{1}$

شرح الحل:

باستعمال معادلة طاقة الحركة نحصل على :

$\ m_{1}(v_{1}^{2}-u_{1}^{2})=m_{2}(u_{2}^{2}-v_{2}^{2})$

$\Rightarrow m_{1}(v_{1}-u_{1})(v_{1}+u_{1})=m_{2}(u_{2}-v_{2})(u_{2}+v_{2})$

وبالنسبة إلى معادلة كمية التحرك نحصل على:

$\ m_{1}(v_{1}-u_{1})=m_{2}(u_{2}-v_{2})$

وبقسمة معادلة طاقة الحركة على معادلة كمية التحرك ، نحصل على:

$\ v_{1}+u_{1}=u_{2}+v_{2}$

$\Rightarrow v_{1}-v_{2}=u_{2}-u_{1}$

ونجد النتائج الآتية:

• تنعكس السرعة النسبية لإحدي الكرات بالنسبة للكرة الأخرى بعد الاصتدام ،

• متوسط كمية تحرك كل كرة قبل وبعد التصادم متساوية .

(هذا مع إهمال قوى الاحتكاك !)

تصادم مرن لجسمين متساويا الكتلة

وكما كان متوقعا ، فالحل لا يتغير إذا قمنا بإضافة ثابت إلى جميع السرعات قبل وبعد الاصتدام . وهذا يعادل إمكانية اختيار مختبر متحرك ( أو مشاهد متحرك ) بسرعة ثابتة ، فهذا لا يؤثر على النتيجة ، وبذلك يتحقق قانوني إنحفاظ طاقة الحركة و إنحفاظ كمية الحركة.

تصادم مرن لجسمين في مختبر متحرك بسرعة ثابتة

 انظر أيضا

• تصادم