دويري

دويري cycloid متولد من دوران دائرة.

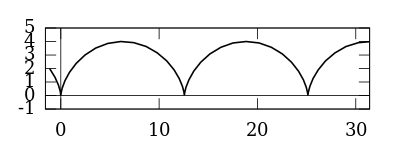
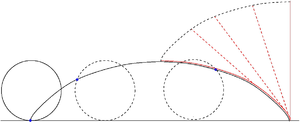
دويري (Cycloid) هو خط منحن تحدثه أيما نقطة من نقاط محيط الدائرة أو الطارة المتدحرجة في سطح مستو. فإذا دار دولاب دراجة هوائية على طريق مستوية استواءً تاماً فإن كل نقطة في المحيط الخارجي للدولاب تشكل دويريا منذ أن تمس الأرض أول مرة إلى أن تعاود مسها من جديد، متممة بذلك دورة كاملة. يكون طول الدويري أربعة أضعاف قطر الدائرة أو الطارة التي أحدثته.

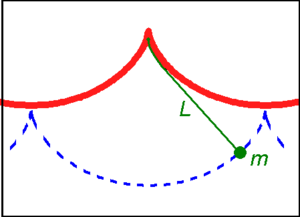
الدويري، بنتوءاته متجهةً لأعلى، هو منحنى أسرع نزول تحت جاذبية ثابتة، وهو أيضاً شكل منحنى تكون فيه فترة نزول غرض على المنحنى لا تعتمد على موضع بداية الغرض.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

المعادلات

دويري مولـَّد من دائرة نصف قطرها

r

= 2

The cycloid through the origin, with a horizontal base given by the line $y$ = 0, generated by a circle of radius $r$ rolling over the "positive" side of the base ( $y$ ≥ 0), consists of the points ( $x$ , $y$ ), with

{\begin{aligned}x&=r(t-\sin t)\\y&=r(1-\cos t)\end{aligned}}

where $t$ is a real parameter, corresponding to the angle through which the rolling circle has rotated, measured in radians. For given $t$ , the circle's centre lies at $x$ = $rt$ , $y$ = $r$ .

Solving for $t$ and replacing, the Cartesian equation is found to be:

x=r\cos ^{-1}\left(1-{\frac {y}{r}}\right)-{\sqrt {y(2r-y)}}.

An equation for the cycloid of the form $y$ = $f$ ( $x$ ) with a closed-form expression for the right-hand side is not possible.

When $y$ is viewed as a function of $x$ , the cycloid is differentiable everywhere except at the cusps, where it hits the $x$ -axis, with the derivative tending toward $\infty$ or $-\infty$ as one approaches a cusp. The map from $t$ to ( $x$ , $y$ ) is a differentiable curve or parametric curve of class $C$ ^∞ and the singularity where the derivative is 0 is an ordinary cusp.

A cycloid segment from one cusp to the next is called an arch of the cycloid. The first arch of the cycloid consists of points such that

0\leq t\leq 2\pi .

The cycloid satisfies the differential equation:

\left({\frac {dy}{dx}}\right)^{2}={\frac {2r}{y}}-1

.

Evolute

Generation of the evolute of the cycloid unwrapping a tense wire placed on half cycloid arc (red marked)

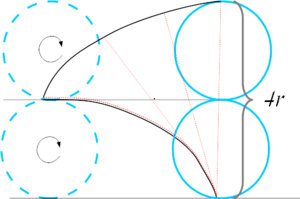
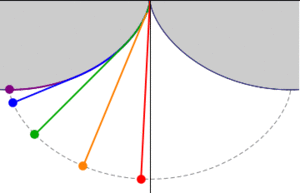
The evolute of the cycloid has the property of being exactly the same cycloid it originates from. This can otherwise be seen from the tip of a wire initially lying on a half arc of cycloid describing a cycloid arc equal to the one it was lying on once unwrapped (see also cycloidal pendulum and arc length).

عرض

عرض لخصائص the evolute لدويري.

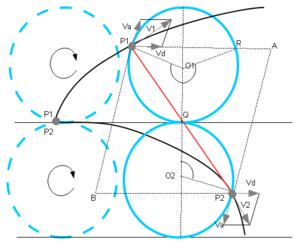
There are several demonstrations of the assertion. The one presented here uses the physical definition of cycloid and the kinematic property that the instantaneous velocity of a point is tangent to its trajectory. Referring to the picture on the right, $P_{1}$ and $P_{2}$ are two tangent points belonging to two rolling circles. The two circles start to roll with same speed and same direction without skidding. $P_{1}$ and $P_{2}$ start to draw two cycloid arcs as in the picture. Considering the line connecting $P_{1}$ and $P_{2}$ at an arbitrary instant (red line), it is possible to prove that the line is anytime tangent in P2 to the lower arc and orthogonal to the tangent in P1 of the upper arc. One sees that:

P1, Q and P2 are aligned because ${\widehat {P1O1Q}}={\widehat {P2O2Q}}$ (equal rolling speed) and therefore ${\widehat {O1QP1}}={\widehat {O2QP2}}$ . Since ${\widehat {O1QP2}}+{\widehat {O2QP2}}=\pi$ by construction, it follows ${\widehat {P1QP2}}=\pi$ .
If A is the meeting point between the perpendicular from P1 to the straight of O1O2 and the tangent to the circle in P2, then the triangle P1AP2 is isosceles because ${\widehat {QP2A}}={\frac {1}{2}}{\widehat {P2O2Q}}$ and ${\widehat {QP1A}}={\frac {1}{2}}{\widehat {QO1R}}=$ (easy to prove seen the construction) $={\frac {1}{2}}{\widehat {QO1P1}}$ . For the previous noted equality between ${\widehat {P1O1Q}}$ and ${\widehat {QO2P2}}$ then ${\widehat {QP1A}}={\widehat {QP2A}}$ and P1AP2 is isosceles.
Conducting from P2 the orthogonal straight to O1O2, from P1 the straight line tangent to the upper circle and calling B the meeting point is now easy to see that P1AP2B is a rhombus, using the theorems concerning the angles between parallel lines
Now consider the speed V2 of P2. It can be seen as the sum of two components, the rolling speed Va and the drifting speed Vd. Both speeds are equal in modulus because the circles roll without skidding. Vd is parallel to P1A and Va is tangent to the lower circle in P2 therefore is parallel to P2A. The total speed of P2, V2, is then parallel to P2P1 because both are diagonals of two rhombuses with parallel sides and has in common with P1P2 the contact point P2. It follows that the speed vector V2 lies on the prolongation of P1P2. Because V2 is tangent to the arc of cycloid in P2, it follows that also P1P2 is tangent.
Analogously, it can be easily demonstrated that P1P2 is orthogonal to V1 (other diagonal of the rhombus).
The tip of an inextensible wire initially stretched on half arc of lower cycloid and bounded to the upper circle in P1 will then follow the point along its path without changing its length because the speed of the tip is at each moment orthogonal to the wire (no stretching or compression). The wire will be at the same time tangent in P2 to the lower arc because the tension and the demonstrated items. If it would not be tangent then there would be a discontinuity in P2 and consequently there would be unbalanced tension forces.

المساحة

إن قوساً واحداً لدويري متولد من من دائرة نصف قطرها r يمكن التعبير عنه بمتغيرات كالتالي:

{\begin{aligned}x&=r(t-\sin t)\\y&=r(1-\cos t)\end{aligned}}

مع ${\frac {dx}{dt}}=r(1-\cos t)$

0\leq t\leq 2\pi .

ولما كان

{\frac {dx}{dt}}=r(1-\cos t)

المساحة تحت القوس هي

{\begin{aligned}A&=\int _{t=0}^{t=2\pi }y\,dx=\int _{t=0}^{t=2\pi }r^{2}(1-\cos t)^{2}dt\\&=\left.r^{2}\left({\frac {3}{2}}t-2\sin t+{\frac {1}{2}}\cos t\sin t\right)\right|_{t=0}^{t=2\pi }\\&=3\pi r^{2}.\end{aligned}}

هذه النتيجة، وبعض التعميمات، يمكن الحصول عليها بدون حسابات باستخدام حسبان مرئي من ماميكون.

طول القوس

The length of the cycloid as consequence of the property of its evolute

The arc length S of one arch is given by

{\begin{aligned}S&=\int _{0}^{2\pi }\left[\left({\frac {\operatorname {d} \!y}{\operatorname {d} \!t}}\right)^{2}+\left({\frac {\operatorname {d} \!x}{\operatorname {d} \!t}}\right)^{2}\right]^{\frac {1}{2}}\operatorname {d} \!t\\&=\int _{0}^{2\pi }r{\sqrt {2-2\cos(t)}}\,\operatorname {d} \!t\\&=\int _{0}^{2\pi }2\,r|\sin {\frac {t}{2}}\ |\operatorname {d} \!t\\&=-4\,r[\cos {\frac {t}{2}}\,]\;from\;t=0\;to\;2\pi \\&=8\,r.\end{aligned}}

Another immediate way to calculate the length of the cycloid given the properties of the evolute is to notice that when a wire describing an evolute has been completely unwrapped it extends itself along two diameters, a length of 4r. Because the wire does not change length during the unwrapping it follows that the length of half an arc of cycloid is 4r and a complete arc is 8r.

البندول الدويري

مخطط للبندول الدويري.

If a simple pendulum is suspended from the cusp of an inverted cycloid, such that the "string" is constrained between the adjacent arcs of the cycloid, and the pendulum's length is equal to that of half the arc length of the cycloid (i.e., twice the diameter of the generating circle), the bob of the pendulum also traces a cycloid path. Such a cycloidal pendulum is isochronous, regardless of amplitude. The equation of motion is given by:

{\begin{aligned}x&=r[\theta (t)-\sin \theta (t)]\\y&=r[\cos \theta (t)-1].\end{aligned}}

Five isochronous cycloidal pendula with different amplitudes.

The 17th-century Dutch mathematician Christiaan Huygens discovered and proved these properties of the cycloid while searching for more accurate pendulum clock designs to be used in navigation.^[1]

المنحنيات ذات الصلة

Several curves are related to the cycloid.

Curtate cycloid: Here the point tracing out the curve is inside the circle, which rolls on a line.
Prolate cycloid: Here the point tracing out the curve is outside the circle, which rolls on a line.
Trochoid: refers to any of the cycloid, the curtate cycloid and the prolate cycloid.
Hypocycloid: The point is on the edge of the circle, which rolls not on a line but on the inside of another circle.
Epicycloid: The point is on the edge of the circle, which rolls not on a line but on the outside of another circle.
Hypotrochoid: As hypocycloid but the point need not be on the edge of its circle.
Epitrochoid: As epicycloid but the point need not be on the edge of its circle.

All these curves are roulettes with a circle rolled along a uniform curvature. The cycloid, epicycloids, and hypocycloids have the property that each is similar to its evolute. If q is the product of that curvature with the circle's radius, signed positive for epi- and negative for hypo-, then the curve:evolute similitude ratio is 1 + 2q.

The classic Spirograph toy traces out hypotrochoid and epitrochoid curves.

الاستخدام في العمارة

Cycloidal arches at the Kimbell Art Museum

The cycloidal arch was used by architect Louis Kahn in his design for the Kimbell Art Museum in Fort Worth, Texas. It was also used in the design of the Hopkins Center in Hanover, New Hampshire.

الاستخدام في تقوس سطح الكمان

Early research indicated that some transverse arching curves of the plates of golden age violins are closely modeled by curtate cycloid curves.^[2] Later work indicates that curtate cycloids do not serve as general models for these curves,^[3] which vary considerably.

. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .

طالع أيضاً

وصلات خارجية

una curva denominata cicloide

هذه بذرة مقالة عن الرياضيات تحتاج للنمو والتحسين، ساهم في إثرائها بالمشاركة في تحريرها.

^ C. Huygens, "The Pendulum Clock or Geometrical Demonstrations Concerning the Motion of Pendula (sic) as Applied to Clocks," Translated by R. J. Blackwell, Iowa State University Press (Ames, Iowa, USA, 1986).
^ Playfair, Q. "Curtate Cycloid Arching in Golden Age Cremonese Violin Family Instruments". Catgut Acoustical Society Journal. II. 4 (7): 48–58.
^ Mottola, RM (2011). "Comparison of Arching Profiles of Golden Age Cremonese Violins and Some Mathematically Generated Curves". Savart Journal. 1 (1).

[1] C. Huygens, "The Pendulum Clock or Geometrical Demonstrations Concerning the Motion of Pendula (sic) as Applied to Clocks," Translated by R. J. Blackwell, Iowa State University Press (Ames, Iowa, USA, 1986).

[2] Playfair, Q. "Curtate Cycloid Arching in Golden Age Cremonese Violin Family Instruments". Catgut Acoustical Society Journal. II. 4 (7): 48–58.

[3] Mottola, RM (2011). "Comparison of Arching Profiles of Golden Age Cremonese Violins and Some Mathematically Generated Curves". Savart Journal. 1 (1).

[1]

[2]

[3]