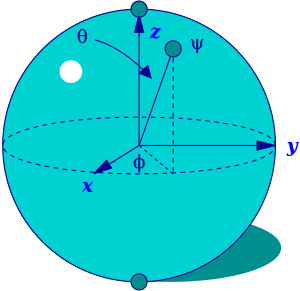
كرة بلوخ

كرة بلوخ

في ميكانيكا وحوسبة الكم، كرة بلوخ هي تمثيل هندسي لفضاء حالة بحتة لـ لنظام بميكانيكا الكم ذي مستويين (كيوبت)، وهي مسماة على اسم الفيزيائي فيلكس بلوخ.

Quantum mechanics is mathematically formulated in Hilbert space or projective Hilbert space. The pure states of a quantum system correspond to the one-dimensional subspaces of the corresponding Hilbert space (or the "points" of the projective Hilbert space). For a two-dimensional Hilbert space, the space of all such states is the complex projective line ℂℙ¹. This is the Bloch sphere, also known to mathematicians as the Riemann sphere.

كرة بلوخ هي a unit 2-sphere, with antipodal points corresponding to a pair of mutually orthogonal state vectors. The north and south poles of the Bloch sphere are typically chosen to correspond to the standard basis vectors خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0\rangle} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1\rangle} , respectively, which in turn might correspond e.g. to the spin-up and spin-down states of an electron. This choice is arbitrary, however. The points on the surface of the sphere correspond to the pure states of the system, whereas the interior points correspond to the mixed states. The Bloch sphere may be generalized to an n-level quantum system, but then the visualization is less useful.

For historical reasons, in optics the Bloch sphere is also known as the كرة پوانكاريه and specifically represents different types of polarizations. Six common polarization types exist and are called Jones vectors. Indeed Henri Poincaré was the first to suggest the use of this kind of geometrical representation at the end of 19th century, as a three-dimensional representation of Stokes parameters.

The natural metric on the Bloch sphere is the Fubini–Study metric. The mapping from the unit 3-sphere in the two-dimensional state space ℂ² to the Bloch sphere is the Hopf fibration, with each ray of spinors mapping to one point on the Bloch sphere.

 التعريف

Given an orthonormal basis, any pure state خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} of a two-level quantum system can be written as a superposition of the basis vectors خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0\rangle} and $|1\rangle$, where the coefficient of (or contribution from) each of the two basis vectors is a complex number. This means that the state is described by four real numbers. However, only the relative phase between the coefficients of the two basis vectors has any physical meaning (the phase of the quantum system is not directly measurable), so that there is redundancy in this description. We can take the coefficient of $|0\rangle$ to be real and non-negative. This allows the state to be described by only three real numbers, giving rise to the three dimensions of the Bloch sphere.

We also know from quantum mechanics that the total probability of the system has to be one:

$\langle \psi |\psi \rangle =1$, or equivalently ${\big \|}|\psi \rangle {\big \|}^{2}=1$.

Given this constraint, we can write خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} using the following representation:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle = \cos\left(\theta /2\right) |0 \rangle \, + \, e^{i\phi} \sin\left(\theta /2\right) |1\rangle = \cos\left(\theta /2\right) |0 \rangle \, + \, (\cos\phi + i\sin\phi) \, \sin\left(\theta /2\right) |1\rangle } , where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0 \leq \theta \leq \pi} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0 \leq \phi < 2 \pi} .

The representation is always unique, because, even though the value of خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi} is not unique when خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} is one of the states (see Bra-ket notation) خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0\rangle} or خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1\rangle} , the point represented by خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi} is unique.

The parameters خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta\,} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi\,} , re-interpreted in spherical coordinates as respectively the colatitude with respect to the z-axis and the longitude with respect to the x-axis, specify a point

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{a} = (\sin\theta \cos\phi,\; \sin\theta \sin\phi,\; \cos\theta) = (u, v, w)}

on the unit sphere in خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^3} .

For mixed states, one considers the density operator. Any two-dimensional density operator ρ can be expanded using the identity I and the Hermitian, traceless Pauli matrices خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{\sigma}} ,

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \rho &= \frac{1}{2}\left(I + \vec{a} \cdot \vec{\sigma}\right) \\ &= \frac{1}{2}\begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix} + \frac{a_x}{2}\begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix} + \frac{a_y}{2}\begin{pmatrix} 0 & -i \\ i & 0 \end{pmatrix} + \frac{a_z}{2}\begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix} \\ &= \frac{1}{2}\begin{pmatrix} 1 + a_z & a_x - ia_y \\ a_x + ia_y & 1 - a_z \end{pmatrix} \end{align}} ,

where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{a} \in \mathbb{R}^3} is called the Bloch vector.

It is this vector that indicates the point within the sphere that corresponds to a given mixed state. Specifically, as a basic feature of the Pauli vector, the eigenvalues of ρ are خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{1}{2}\left(1 \pm |\vec{a}|\right)} . Density operators must be positive-semidefinite, so it follows that خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\vec{a}\right| \le 1} .

For pure states, one then has

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \operatorname{tr}\left(\rho^2\right) = \frac{1}{2}\left(1 + \left|\vec{a}\right|^2 \right) = 1 \quad \Leftrightarrow \quad \left|\vec{a}\right| = 1 ~,}

in comportance with the above.

As a consequence, the surface of the Bloch sphere represents all the pure states of a two-dimensional quantum system, whereas the interior corresponds to all the mixed states.

 u, v, w representation

The Bloch vector خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{a} = (u,v,w)} can be represented in the following basis, with reference to the density operator خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho} :

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u = \rho_{10} + \rho_{01} = 2 \operatorname{Re}(\rho_{01})}

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle v = i(\rho_{01} - \rho_{10}) = 2 \operatorname{Im}(\rho_{10})}

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle w = \rho_{00} - \rho_{11}}

where

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho = \begin{pmatrix} \rho_{00} & \rho_{01} \\ \rho_{10} & \rho_{11} \end{pmatrix} = \frac{1}{2}\begin{pmatrix} 1+w & u-iv \\ u+iv & 1-w \end{pmatrix}. }

This basis is often used in laser theory, where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle w} is known as the population inversion. In this basis, the values خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u, v, w} are the expectations of the three Pauli matrices خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle X, Y, Z} , allowing one to identify the three coordinates with x y and z axes.

 Pure states

Consider an n-level quantum mechanical system. This system is described by an n-dimensional Hilbert space H_n. The pure state space is by definition the set of rays of H_n.

Theorem. Let U(n) be the Lie group of unitary matrices of size n. Then the pure state space of H_n can be identified with the compact coset space

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \operatorname{U}(n) /(\operatorname{U}(n - 1) \times \operatorname{U}(1)). }

To prove this fact, note that there is a natural group action of U(n) on the set of states of H_n. This action is continuous and transitive on the pure states. For any state خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} , the isotropy group of خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} , (defined as the set of elements خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle g} of U(n) such that خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle g |\psi\rangle = |\psi\rangle} ) is isomorphic to the product group

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \operatorname{U}(n - 1) \times \operatorname{U}(1). }

In linear algebra terms, this can be justified as follows. Any خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle g} of U(n) that leaves خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} invariant must have خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} as an eigenvector. Since the corresponding eigenvalue must be a complex number of modulus 1, this gives the U(1) factor of the isotropy group. The other part of the isotropy group is parametrized by the unitary matrices on the orthogonal complement of خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi\rangle} , which is isomorphic to U(n − 1). From this the assertion of the theorem follows from basic facts about transitive group actions of compact groups.

The important fact to note above is that the unitary group acts transitively on pure states.

Now the (real) dimension of U(n) is n². This is easy to see since the exponential map

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle A \mapsto e^{i A} }

is a local homeomorphism from the space of self-adjoint complex matrices to U(n). The space of self-adjoint complex matrices has real dimension n².

Corollary. The real dimension of the pure state space of H_n is 2n − 2.

In fact,

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle n^2 - \left((n - 1)^2 + 1\right) = 2n - 2. \quad }

Let us apply this to consider the real dimension of an m qubit quantum register. The corresponding Hilbert space has dimension 2^m.

Corollary. The real dimension of the pure state space of an m-qubit quantum register is 2^m+1 − 2.

 Plotting pure two-spinor states through stereographic projection

ملف:Riemann Spin2States.jpg

Bloch sphere centered at the origin of خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^3} . A pair of points on it, خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\uparrow\right\rangle} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\downarrow\right\rangle} have been chosen as a basis. Mathematically they are orthogonal even though graphically the angle between them is π. In خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^3} those points have coordinates (0,0,1) and (0,0,−1). An arbitrary spinor خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\nearrow\right\rangle} on the Bloch sphere is representable as a unique linear combination of the two basis spinors, with coefficients being a pair of complex numbers; call them α and β. Let their ratio be خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u = {\beta \over \alpha}} , which is also a complex number خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_x + i u_y} . Consider the plane z = 0, the equatorial plane of the sphere, as it were, to be a complex plane and that the point u is plotted on it as خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle (u_x, u_y, 0)} . Project point u stereographically onto the Bloch sphere away from the South Pole — as it were — (0,0,−1). The projection is onto a point marked on the sphere as خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\nearrow\right\rangle} .

Mathematically the Bloch sphere for a two-spinor state can be mapped to a Riemann sphere خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{C}\mathbf{P}^1} , i.e., the projective Hilbert space خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{P}(H_2)} with the 2-dimensional complex Hilbert space خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_2} a representation space of SO(3). Given a pure state

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha \left|\uparrow \right\rangle + \beta \left|\downarrow \right\rangle = \left|\nearrow \right\rangle }

where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \beta} are complex numbers which are normalized so that

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\alpha|^2 + |\beta|^2 = \alpha^* \alpha + \beta^* \beta = 1}

and such that خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \langle\downarrow | \uparrow\rangle = 0} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \langle\downarrow | \downarrow\rangle = \langle\uparrow | \uparrow\rangle = 1} , i.e., such that خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\uparrow\right\rangle} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\downarrow\right\rangle} form a basis and have diametrically opposite representations on the Bloch sphere, then let

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u = {\beta \over \alpha} = {\alpha^* \beta \over \alpha^* \alpha} = {\alpha^* \beta \over |\alpha|^2} = u_x + i u_y}

be their ratio.

If the Bloch sphere is thought of as being embedded in خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^3} with its center at the origin and with radius one, then the plane z = 0 (which intersects the Bloch sphere at a great circle; the sphere's equator, as it were) can be thought of as an Argand diagram. Plot point u in this plane — so that in خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^3} it has coordinates خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle (u_x, u_y, 0)} .

Draw a straight line through u and through the point on the sphere that represents خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\downarrow\right\rangle} . (Let (0,0,1) represent خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\uparrow\right\rangle} and (0,0,−1) represent خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\downarrow\right\rangle} .) This line intersects the sphere at another point besides خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\downarrow\right\rangle} . (The only exception is when خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle u = \infty} , i.e., when خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha = 0} and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \beta \ne 0} .) Call this point P. Point u on the plane z = 0 is the stereographic projection of point P on the Bloch sphere. The vector with tail at the origin and tip at P is the direction in 3-D space corresponding to the spinor خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\nearrow\right\rangle} . The coordinates of P are

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle P_x = {2 u_x \over 1 + u_x^2 + u_y^2},}

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle P_y = {2 u_y \over 1 + u_x^2 + u_y^2},}

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle P_z = {1 - u_x^2 - u_y^2 \over 1 + u_x^2 + u_y^2}.}

 Density operators

Formulations of quantum mechanics in terms of pure states are adequate for isolated systems; in general quantum mechanical systems need to be described in terms of density operators. The Bloch sphere parametrizes not only pure states but mixed states for 2-level systems. The density operator describing the mixed-state of a 2-level quantum system (qubit) corresponds to a point inside the Bloch sphere with the following coordinates:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left( \sum p_i x_i, \sum p_i y_i, \sum p_i z_i \right),}

where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle p_i} is the probability of the individual states within the ensemble and خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_i, y_i, z_i} are the coordinates of the individual states (on the surface of Bloch sphere). The set of all points on and inside the Bloch sphere is known as the Bloch ball.

For states of higher dimensions there is difficulty in extending this to mixed states. The topological description is complicated by the fact that the unitary group does not act transitively on density operators. The orbits moreover are extremely diverse as follows from the following observation:

Theorem. Suppose A is a density operator on an n level quantum mechanical system whose distinct eigenvalues are μ₁, ..., μ_k with multiplicities n₁, ..., n_k. Then the group of unitary operators V such that V A V* = A is isomorphic (as a Lie group) to

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \operatorname{U}(n_1) \times \cdots \times \operatorname{U}(n_k).}

In particular the orbit of A is isomorphic to

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \operatorname{U}(n)/\left(\operatorname{U}(n_1) \times \cdots \times \operatorname{U}(n_k)\right).}

It is possible to generalize the construction of the Bloch ball to dimensions larger than 2, but the geometry of such a "Bloch body" is more complicated than that of a ball.

 Rotations

A useful advantage of the Bloch sphere representation is that the evolution of the qubit state is describable by rotations of the Bloch sphere. The most concise explanation for why this is the case is that the Lie algebra for the group of unitary and hermitian matrices خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle SU(2)} is isomorphic to the Lie algebra of the group of three dimensional rotations خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle SO(3)} .

 Rotation operators about the Bloch basis

The rotations of the Bloch sphere about the Cartesian axes in the Bloch basis are given by

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} R_x(\theta) &= e^{(-i \theta X/2)} = \cos(\theta /2)I - i\sin(\theta/2)X = \begin{bmatrix} \cos \theta/2 & -i \sin \theta/2 \\ -i \sin \theta/2 & \cos \theta/2 \end{bmatrix} \\ R_y(\theta) &= e^{(-i \theta Y/2)} = \cos(\theta /2)I - i\sin(\theta/2)Y = \begin{bmatrix} \cos \theta/2 & -\sin \theta/2 \\ \sin \theta/2 & \cos \theta/2 \end{bmatrix} \\ R_z(\theta) &= e^{(-i \theta Z/2)} = \cos(\theta /2)I - i\sin(\theta/2)Z = \begin{bmatrix} e^{-i \theta/2} & 0 \\ 0 & e^{i \theta/2} \end{bmatrix} \end{align}}

 الدورانات حول محور عام

If خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat{n} = (n_x, n_y, n_z) } is a real unit vector in three dimensions, the rotation of the Bloch sphere about this axis is given by:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle R_{\hat{n}}(\theta) = \exp\left(-i\theta\hat{n} \cdot \frac{1}{2}\vec{\sigma}\right) }

An interesting thing to note is that this expression is identical under relabelling to the extended Euler formula for pure imaginary quaternions.

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{q} = e^{\frac{1}{2}\theta(u_x\mathbf{i} + u_y\mathbf{j} + u_z\mathbf{k})} = \cos \frac{\theta}{2} + (u_x\mathbf{i} + u_y\mathbf{j} + u_z\mathbf{k}) \sin \frac{\theta}{2} }

 اشتقاق مولد دوران بلوخ

Ballentine presents an intuitive derivation for the infinitesimal unitary transformation. This is important for understanding why the rotations of Bloch spheres are exponentials of linear combinations of Pauli matrices. Hence a brief treatment on this is given here. A more complete description in a quantum mechanical context can be found here.

Consider a family of unitary operators خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U} representing a rotation about some axis. Since the rotation has one degree of freedom, the operator acts on a field of scalars خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle S} such that:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U(0) = I }

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U(s_1 + s_2) = U(s_1)U(s_2) }

where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0, s_1, s_2, \in S }

We define the infinitesimal unitary as the Taylor expansion truncated at second order.

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U(s) = I + \frac{dU}{ds} \Bigg|_{s=0} s + O\left(s^2\right) }

By the unitary condition:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U^{\dagger}U = I }

Hence

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U^{\dagger}U = I + s\left(\frac{dU}{ds}\Bigg|_{s=0} + \frac{dU^{\dagger}}{ds}\Bigg|_{s=0}\right) + O\left(s^2\right) = I }

For this equality to hold true (assuming خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle O\left(s^2\right)} is negligible) we require

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{dU}{ds} \Bigg|_{s=0} + \frac{dU^{\dagger}}{ds} \Bigg|_{s=0}= 0} .

This results in a solution of the form:

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{dU}{ds} \Bigg|_{s=0} = iK }

where خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle K} is any Hermitian transformation, and is called the generator of the unitary family. Hence

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle U(s) = e^{iKs} }

Since the Pauli matrices خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle (\sigma_x, \sigma_y, \sigma_z)} are unitary Hermitian matrices and have eigenvectors corresponding to the Bloch basis, خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle (\hat{x}, \hat{y}, \hat{z})} , we can naturally see how a rotation of the Bloch sphere about an arbitrary axis خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat{n}} is described by

خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle R_{\hat{n}}(\theta) = \exp(-i \theta \hat{n} \cdot \vec{\sigma}/2) }

with the rotation generator given by خطأ رياضيات (اعرض بصيغة MathML إن أمكن (تحت التجريب): رد غير صحيح ("Math extension cannot connect to Restbase.") من الخادم "https://wikimedia.org/api/rest_v1/":): {\displaystyle K = \hat{n} \cdot \vec{\sigma}/2. }

 انظر أيضاً

• Specific implementations of the Bloch sphere are enumerated under the qubit article.
• Atomic electron transition
• Gyrovector space
• Versors

 وصلات خارجية

• Online Bloch sphere visualization by Konstantin Herb